Clarence Barlow: Pinball Play (2010)

for 4 Bohlen-Pierce clarinets

On Pinball Play

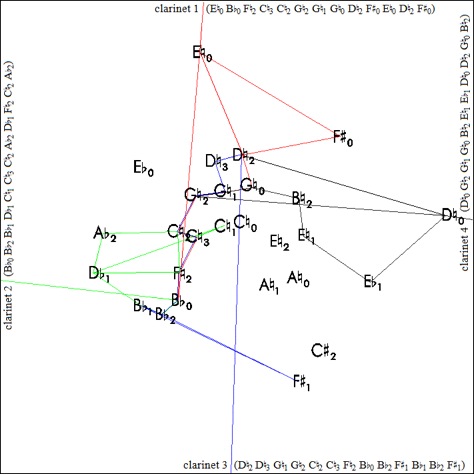
In 1978 I developed a method for quantitatively establishing the harmonicity of an interval of pitch, given the relationship of its frequencies as a ratio. For instance, according to this system, the 2:3 perfect fifth is 0.273 harmonic, the 4:5 major third 0.119 etc. This method can convert a scale given in cents from a designated tonic into ratios related to that tonic. The intrascalar intervals’ harmonicities can then be multi-dimensionally scaled, as in the square-shaped map below: the more harmonic the relationship of two given pitches, the closer together they are in the map.

This diagram shows the multi-dimensional scaling of the rationalized Bohlen-Pierce (“BP”) scale spanning two adjacent perfect twelfths. As the main part of the compositional process, four straight lines are repeatedly projected into the square, each from a different side. If a line meets one of the notes of the scale, the note is sounded and the line is generally deflected to a spatially nearby note, which is also sounded. This process is repeated until a path is re-traversed or the line exits from the diagram. These constantly varying trajectories, not unlike those in a pinball game, generate melodies distributed among four clarinets, one for each side of the diagram. The four clarinets repeatedly play a new melody starting at multiples of 4″, 3″, 3½” and 2½” respectively. The melodies initially have a length of about half a second but gradually get longer. The diagram also shows the final four melodies of the piece.

The word “Play” in the title, a play on words, refers to the obvious “game” and “dramaturgy” and indirectly to my melodic “prey” or “catch”, which is “prooi” in Dutch (the BP scale was co-invented by Kees van Prooijen).

Download score to Pinball Play